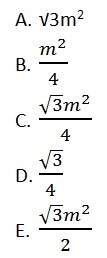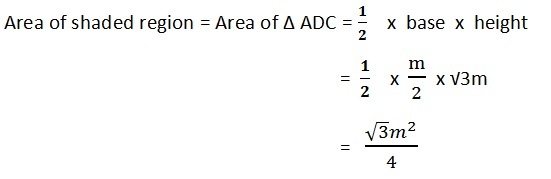Triangle Review: Fundamentals of Triangle , Formula’s for Triangle , Types of Triangle , 45° – 45° – 90° Triangle , 30° – 60° – 90° Triangle , Congruent Triangles, Similar Triangles
Class Questions : Practice Examples 1, Practice Examples 2
Practice Questions : Exercise 1, Exercise 2, Exercise 3 , Exercise 4
Q.11
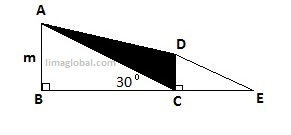
In below figure what is the area of the shaded region if CD is half of AB?
Solution;
Δ ABC is a 30° – 60° – 90° triangle, so the length of sides are in the ratio of 1: √3: 2.
If we draw some more lines, we will get following figure.
Now it is easier to find the area of the shaded region.
The shaded region is a triangle ADC.
Height = AO = BC = √3m
Base DC = ½ x m
= m/2
Correct answer choice is ‘C’.
Q.12
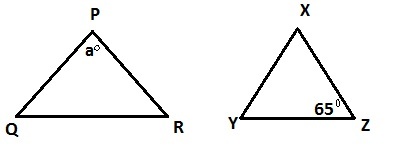
In the above figure PQ = XY, PR = XZ, QR = YZ and XY = XZ then what is the value of ‘a’?
A. 65°
B. 55°
C. 40°
D. 50°
E. 120°
Solution;
As given in the question PQ = XY, PR = XZ and QR =YZ that means both are congruent triangles. Here it is also given that XY = XZ which defines
Δ XYZ as an Isosceles triangle.
In Δ XYZ; ∠ Z = 65°, ∠ Y will also be = 65° then ∠ X = 180° – 65° – 65°
∠ X = 50°
In congruent triangles ∠P = ∠X, the value of a = 50°.
Correct answer choice is ‘D’.
<<<< PREVIOUS PAGE >>>>>>>>>NEXT PAGE
Triangle Review: Fundamentals of Triangle , Formula’s for Triangle , Types of Triangle , 45° – 45° – 90° Triangle , 30° – 60° – 90° Triangle , Congruent Triangles, Similar Triangles
Class Questions : Practice Examples 1, Practice Examples 2
Practice Questions : Exercise 1, Exercise 2, Exercise 3 , Exercise 4