Reveiw : Percent Increase and Decrease , Cumulative Percent , Simple and Compound Interest
Practice Examples 1, Practice Examples 2
Percent Increase and Decrease
Percent Increase – When the quantity increase from starting initial value is called as Percent Increase. An increase of 100% in a quantity means 100 % + 100 % = 200% of initial amount. Similarly an increase of 500 % is total 100% + 500 % = 600 % of initial amount.
Example 1 : The price of laptop increases from $500 to $525 , Find the change in price in terms of percent?
Answer:
Original Value = $500 , New Value $ 525 So, there is $ 25 increase in price
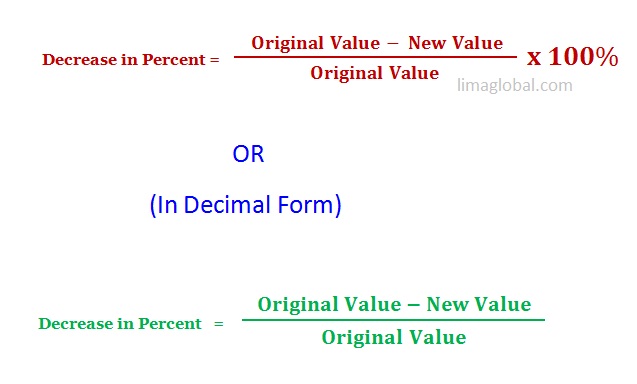
Percent Decrease – When the quantity decreases from starting initial value is known as Percent Decrease. The decrease of 30% in a quantity means the final value will be 70% of initial value. Like if water level in a tank decreased by 25% that means now it will have 100% – 25% = 75% water in the tank.
Example 2 :
In a boiler of 5000 liters capacity, 20 % water is evaporated. Find out how much water is left.
Answer : Initial amount = 5000 liters = 100% ,
Water evaporated = 20 %
Water left = Decrease in Water = (100 – 20) /100
= 0.80
So , 0.80 of 5000 liters = 0.80 x 5000 = 4000 liters
Cumulative or Successive Percent Change
In cumulative percent, Percent Changes more than one time from the original value.
Like for example there is increase of 2% in price of Petrol and after one week there is decrease of 9% in price of petrol. Total what percent of change occurred in price of petrol?
Solution;
Solve this type of sums by converting the percent in decimal equivalents.
Let ‘M’ be the original price , So increase in price will be 102% The Decimal Equivalent of 102 % is 1.02.
After one week price of petrol decreases by 9% So new price of petrol will 91% of increased price. Decimal Equivalent of 91% will be 0.91.
So Successive or cumulative percent change in price of petrol after one week will be;
(1.02) x (0.91) x M
= 0.9282 M
Percent Equivalent of 0.9282 = 92.82%. As it is lesser than 100%, there will a cumulative decrease in price by 100 – 92.82 = 7.18% from the original price after one week.
OR
Another way to solve this sum is by taking the original price of petrol =$100.
First there is an increase of 2 %; so price of petrol is $102
After one week price decrease by 9 %, i.e. new price is 91% of $102
New Price = (91/100) x 102
= $ 92.82
As the new price is below $100 ,So after one week the price of the petrol will be lesser by
$100 – $92.82 = $ 7.18.