Reveiw : Percent Increase and Decrease , Cumulative Percent , Simple and Compound Interest
Practice Examples 1, Practice Examples 2
Percent is used to represent ratios of part to whole where whole is 100. It is represented with symbol “ %” that means divided by 100 or 1/100.
Fraction Equivalent to Percent
30 % = 30 x 1/100 = 3/10
25% = 25 x 1 / 100 = 1/4
50 % = 50 x 1/100 = 1/2
100 % = 100 x 1/100 = 1
1 % = 1 x 1/100 = 1/100
0.25% = 0.25 x 1/100 = 0.25/100
0.50 % = 0.50 x 1/100 = 0.50/100
0.01% = 0.01 x 1/100 = 0.01/100
Decimal Equivalent to Percent
To convert the Percent to Decimal , put a decimal mark ahead of two digits of percent.
- 100 % = 1.00
- 90 % = 0.90
- 80 % = 0.80
- 68% = 0.68
- 25% = 0.25
- 1 % = 1 x 1/100 = 1/100 = 0.01
- 0.25% = 0.25 x 1/100 = 0.0025
- 0.50 % = 0.50 x 1/100 = 0.0050
- 0.01% = 0.01 x 1/100 = 0.0001
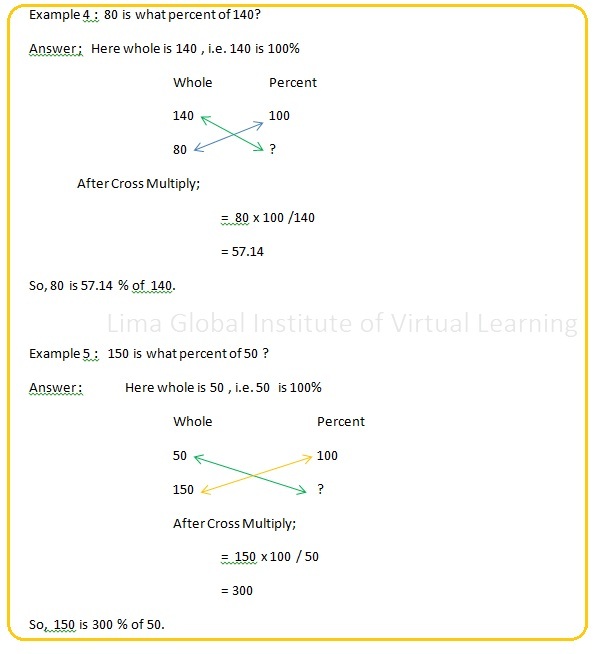
Finding percent of Part:
Now, we will be given part of a whole and we have to find out that this part is what percent of whole.
Example 6 : 42 is 70% of what number?
Answer ; Here we have to find the whole ,
Percent Whole
70 42
100 ?
After cross multiplying we will get
= 100 x 42 / 70
= 60
So 42 is 70% of 60.
Base Value
The initial amount of any quantity is the base value.
Percent Change
When the quantity changes from its initial value it is being referred as “Percent Change” ; like water in a tank used , Salary of a person changed, Interest rates on savings etc.
Percent Increase – When the quantity increase from starting initial value is called as Percent Increase. An increase of 100% in a quantity means 100 % + 100 % = 200% of initial amount. Similarly an increase of 500 % is total 100% + 500 % = 600 % of initial amount.
Percent Decrease – When the quantity decreases from starting initial value is known as Percent Decrease . The decrease of 30% in a quantity means the final value will be 70% of initial value. Like if water level in a tank decreased by 25% that means now it will have 100% – 25% = 75% water in the tank.